
- school Campus Bookshelves
- menu_book Bookshelves
- perm_media Learning Objects
- login Login
- how_to_reg Request Instructor Account
- hub Instructor Commons

Margin Size
- Download Page (PDF)
- Download Full Book (PDF)
- Periodic Table
- Physics Constants
- Scientific Calculator
- Reference & Cite
- Tools expand_more
- Readability
selected template will load here
This action is not available.

12.2: Exercises
- Last updated
- Save as PDF
- Page ID 52784

- Laird C. Sheldahl
- Mt. Hood Community College
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
LAB 12 EXERCISES \(\PageIndex{1}\)
Lab 12 exercises \(\pageindex{2}\), lab 12 exercises \(\pageindex{3}\), spinal cord and nerve anatomy, lab 12 exercises \(\pageindex{4}\), vertebral column.
Match these items to their relative position: Cervical enlargement, Lumbar enlargement*, Conus medullaris, Cauda equina, Filum terminale ( * trickier than you might expect)
LAB 12 EXERCISES \(\PageIndex{5}\)
Peripheral nerves and plexuses, lab 12 exercises \(\pageindex{6}\), draw your own cranial nerve study sheet.
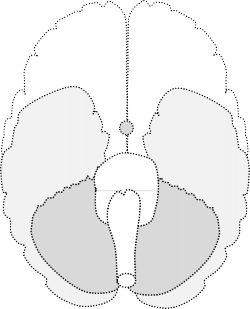

Matching Activity

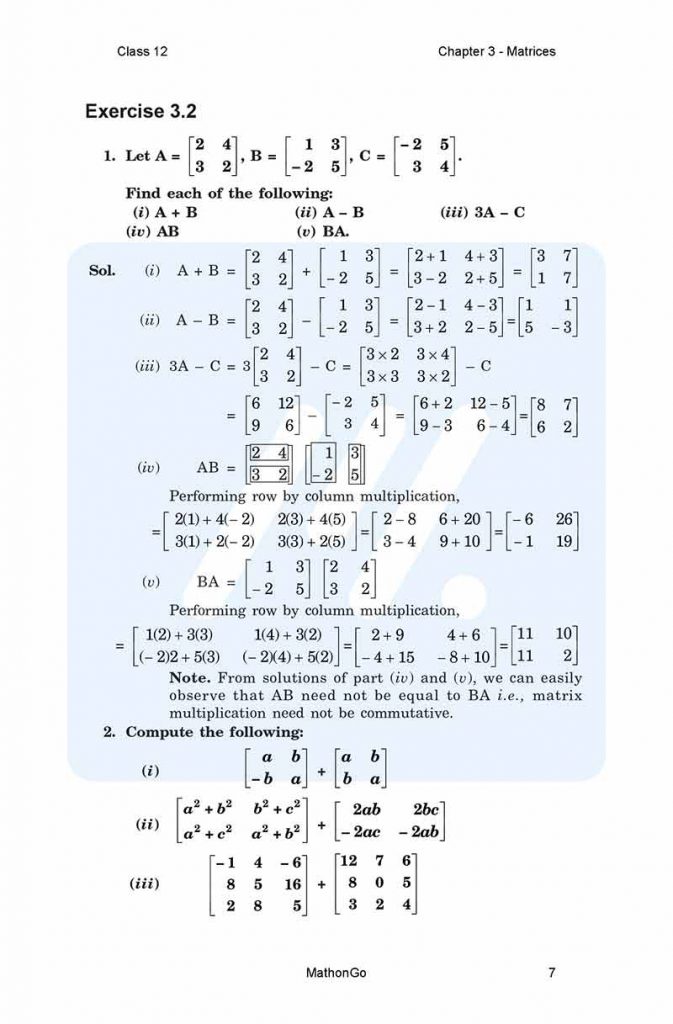
NCERT Solutions for Class 12 Maths Chapter 3 Exercise 3.2
Ncert solutions for class 12 maths chapter 3 matrices ex 3.2.
Free PDF of NCERT Solutions for Class 12 Maths Chapter 3 Exercise 3.2 prepared by expert Mathematics teacher at Mathongo.com as per CBSE (NCERT) books guidelines. Download our Class 12 Maths Chapter 3 Matrices Ex 3.2 Questions with Solutions to help you to revise complete Syllabus and Score More marks in your exams.
Share with friends:

- school Campus Bookshelves
- menu_book Bookshelves
- perm_media Learning Objects
- login Login
- how_to_reg Request Instructor Account
- hub Instructor Commons
Margin Size
- Download Page (PDF)
- Download Full Book (PDF)
- Periodic Table
- Physics Constants
- Scientific Calculator
- Reference & Cite
- Tools expand_more
- Readability
selected template will load here
This action is not available.

12.3E: Exercises
- Last updated
- Save as PDF
- Page ID 30578

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
Practice Makes Perfect
Exercise \(\pageindex{17}\) determine if a sequence is arithmetic.
In the following exercises, determine if each sequence is arithmetic, and if so, indicate the common difference.
- \(4,12,20,28,36,44, \dots\)
- \(-7,-2,3,8,13,18, \dots\)
- \(-15,-16,3,12,21,30, \dots\)
- \(11,5,-1,-7-13,-19, \dots\)
- \(8,5,2,-1,-4,-7, \dots\)
- \(15,5,-5,-15,-25,-35, \dots\)
1. The sequence is arithmetic with common difference \(d=8\).
3. The sequence is not arithmetic.
5. The sequence is arithmetic with common difference \(d=−3\).
Exercise \(\PageIndex{18}\) Determine if a Sequence is Arithmetic
In the following exercises, write the first five terms of each sequence with the given first term and common difference.
- \(a_{1}=11\) and \(d=7\)
- \(a_{1}=18\) and \(d=9\)
- \(a_{1}=-7\) and \(d=4\)
- \(a_{1}=-8\) and \(d=5\)
- \(a_{1}=14\) and \(d=-9\)
- \(a_{1}=-3\) and \(d=-3\)
1. \(11,18,25,32,39\)
3. \(-7,-3,1,5,9\)
5. \(14,5,-4,-13,-22\)
Exercise \(\PageIndex{19}\) Find the General Term (\(n\)the Term) of an Arithmetic Sequence
In the following exercises, find the term described using the information provided.
- Find the twenty-first term of a sequence where the first term is three and the common difference is eight.
- Find the twenty-third term of a sequence where the first term is six and the common difference is four.
- Find the thirtieth term of a sequence where the first term is \(−14\) and the common difference is five.
- Find the fortieth term of a sequence where the first term is \(−19\) and the common difference is seven.
- Find the sixteenth term of a sequence where the first term is \(11\) and the common difference is \(−6\).
- Find the fourteenth term of a sequence where the first term is eight and the common difference is \(−3\).
- Find the twentieth term of a sequence where the fifth term is \(−4\) and the common difference is \(−2\). Give the formula for the general term.
- Find the thirteenth term of a sequence where the sixth term is \(−1\) and the common difference is \(−4\). Give the formula for the general term.
- Find the eleventh term of a sequence where the third term is \(19\) and the common difference is five. Give the formula for the general term.
- Find the fifteenth term of a sequence where the tenth term is \(17\) and the common difference is seven. Give the formula for the general term.
- Find the eighth term of a sequence where the seventh term is \(−8\) and the common difference is \(−5\). Give the formula for the general term.
- Find the fifteenth term of a sequence where the tenth term is \(−11\) and the common difference is \(−3\). Give the formula for the general term.
7. \(a_{20}=-34 .\) The general term is \(a_{n}=-2 n+6\).
9. \(a_{11}=59 .\) The general term is \(a_{n}=5 n+4\).
11. \(a_{8}=-13 .\) The general term is \(a_{n}=-5 n+27\).
Exercise \(\PageIndex{20}\) Find the General Term (\(n\)the Term) of an Arithmetic Sequence
In the following exercises, find the first term and common difference of the sequence with the given terms. Give the formula for the general term.
- The second term is \(14\) and the thirteenth term is \(47\).
- The third term is \(18\) and the fourteenth term is \(73\).
- The second term is \(13\) and the tenth term is \(−51\).
- The third term is four and the tenth term is \(−38\).
- The fourth term is \(−6\) and the fifteenth term is \(27\).
- The third term is \(−13\) and the seventeenth term is \(15\).
1. \(a_{1}=11, d=3 .\) The general term is \(a_{n}=3 n+8\).
3. \(a_{1}=21, d=-8 .\) The general term is \(a_{n}=-8 n+29\)
5. \(a_{1}=-15, d=3 .\) The general term is \(a_{n}=3 n-18\).

Exercise \(\PageIndex{21}\) Find the Sum of the First \(n\) Terms of an Arithmetic Sequence
In the following exercises, find the sum of the first \(30\) terms of each arithmetic sequence.
- \(11,14,17,20,23, \dots\)
- \(12,18,24,30,36, \dots\)
- \(8,5,2,-1,-4, \dots\)
- \(16,10,4,-2,-8, \dots\)
- \(-17,-15,-13,-11,-9, \dots\)
- \(-15,-12,-9,-6,-3, \dots\)
1. \(1,635\)
3. \(-1,065\)
Exercise \(\PageIndex{22}\) Find the Sum of the First \(n\) Terms of an Arithmetic Sequence
In the following exercises, find the sum of the first \(50\) terms of the arithmetic sequence whose general term is given.
- \(a_{n}=5 n-1\)
- \(a_{n}=2 n+7\)
- \(a_{n}=-3 n+5\)
- \(a_{n}=-4 n+3\)
1. \(6,325\)
3. \(-3,575\)
Exercise \(\PageIndex{23}\) Find the Sum of the First \(n\) Terms of an Arithmetic Sequence
In the following exercises, find each sum.
- \(\sum_{i=1}^{40}(8 i-7)\)
- \(\sum_{i=1}^{45}(7 i-5)\)
- \(\sum_{i=1}^{50}(3 i+6)\)
- \(\sum_{i=1}^{25}(4 i+3)\)
- \(\sum_{i=1}^{35}(-6 i-2)\)
- \(\sum_{i=1}^{30}(-5 i+1)\)
1. \(6,280\)
3. \(4,125\)
5. \(-3,580\)
Exercise \(\PageIndex{24}\) Writing Exercises
- In your own words, explain how to determine whether a sequence is arithmetic.
- In your own words, explain how the first two terms are used to find the tenth term. Show an example to illustrate your explanation.
- In your own words, explain how to find the general term of an arithmetic sequence.
- In your own words, explain how to find the sum of the first \(n\) terms of an arithmetic sequence without adding all the terms.
1. Answer may vary
3. Answer may vary
a. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
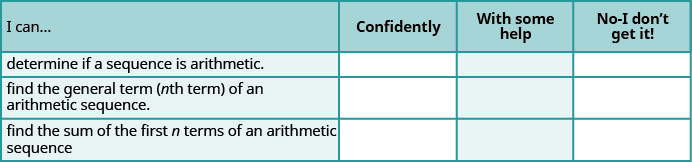
b. After reviewing this checklist, what will you do to become confident for all objectives?

- school Campus Bookshelves
- menu_book Bookshelves
- perm_media Learning Objects
- login Login
- how_to_reg Request Instructor Account
- hub Instructor Commons
Margin Size
- Download Page (PDF)
- Download Full Book (PDF)
- Periodic Table
- Physics Constants
- Scientific Calculator
- Reference & Cite
- Tools expand_more
- Readability
selected template will load here
This action is not available.

12.5: The Matching Problem
- Last updated
- Save as PDF
- Page ID 10248

- Kyle Siegrist
- University of Alabama in Huntsville via Random Services
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
Definitions and Notation
The matching experiment.
The matching experiment is a random experiment that can the formulated in a number of colorful ways:
- Suppose that \(n\) male-female couples are at a party and that the males and females are randomly paired for a dance. A match occurs if a couple happens to be paired together.
- An absent-minded secretary prepares \(n\) letters and envelopes to send to \(n\) different people, but then randomly stuffs the letters into the envelopes. A match occurs if a letter is inserted in the proper envelope.
- \(n\) people with hats have had a bit too much to drink at a party. As they leave the party, each person randomly grabs a hat. A match occurs if a person gets his or her own hat.
These experiments are clearly equivalent from a mathematical point of view, and correspond to selecting a random permutation \(\bs{X} = (X_1, X_2, \ldots, X_n)\) of the population \(D_n = \{1, 2, \ldots, n\}\). Here are the interpretations for the examples above:
- Number the couples from 1 to \(n\). Then \(X_i\) is the number of the woman paired with the \(i\)th man.
- Number the letters and corresponding envelopes from 1 to \(n\). Then \(X_i\) is the number of the envelope containing the \(i\)th letter.
- Number the people and their corresponding hats from 1 to \(n\). Then \(X_i\) is the number of the hat chosen by the \(i\)th person.
Our modeling assumption, of course, is that \(\bs{X}\) is uniformly distributed on the sample space of permutations of \(D_n\). The number of objects \(n\) is the basic parameter of the experiment. We will also consider the case of sampling with replacement from the population \(D_n\), because the analysis is much easier but still provides insight. In this case, \(\bs{X}\) is a sequence of independent random variables, each uniformly distributed over \(D_n\).
We will say that a match occurs at position \(j\) if \(X_j = j\). Thus, number of matches is the random variable \(N\) defined mathematically by \[ N_n = \sum_{j=1}^n I_j\] where \(I_j = \bs{1}(X_j = j)\) is the indicator variable for the event of match at position \(j\). Our problem is to compute the probability distribution of the number of matches. This is an old and famous problem in probability that was first considered by Pierre-Remond Montmort; it sometimes referred to as Montmort's matching problem in his honor.
Sampling With Replacement
First let's solve the matching problem in the easy case, when the sampling is with replacement. Of course, this is not the way that the matching game is usually played, but the analysis will give us some insight.
\((I_1, I_2, \ldots, I_n)\) is a sequence of \(n\) Bernoulli trials, with success probability \(\frac{1}{n}\).
The variables are independent since the sampling is with replacement. Since \(X_j\) is uniformly distributed, \(\P(I_j = 1) = \P(X_j = j) = \frac{1}{n}\).
The number of matches \(N_n\) has the binomial distribution with trial parameter \(n\) and success parameter \(\frac{1}{n}\). \[ \P(N_n = k) = \binom{n}{k} \left(\frac{1}{n}\right)^k \left(1 - \frac{1}{n}\right)^{n-k}, \quad k \in \{0, 1, \ldots, n\} \]
This follows immediately from the previous result on Bernoulli trials .
The mean and variance of the number of matches are
- \(\E(N_n) = 1\)
- \(\var(N_n) = \frac{n-1}{n}\)
These results follow from the previous result on the binomial distribution of \( N_n \). Recall that the binomial distribution with parameters \(n\) and \(p\) has mean \(n p\) and variance \(n p (1 - p)\).
The distribution of the number of matches converges to the Poisson distribution with parameter 1 as \(n \to \infty\): \[ \P(N_n = k) \to \frac{e^{-1}}{k!} \text{ as } n \to \infty \text{ for } k \in \N \]
This is a special case of the convergence of the binomial distribution to the Poisson. For a direct proof, note that \[ \P(N_n = k) = \frac{1}{k!} \frac{n^{(k)}}{n^k} \left(1 - \frac{1}{n}\right)^{n-k} \] But \(\frac{n^{(k)}}{n^k} \to 1\) as \(n \to \infty\) and \(\left(1 - \frac{1}{n}\right)^{n-k} \to e^{-1}\) as \(n \to \infty\) by a famous limit from calculus.
Sampling Without Replacement
Now let's consider the case of real interest, when the sampling is without replacement, so that \(\bs{X}\) is a random permutation of the elements of \(D_n = \{1, 2, \ldots, n\}\).
Counting Permutations with Matches
To find the probability density function of \(N_n\), we need to count the number of permutations of \(D_n\) with a specified number of matches. This will turn out to be easy once we have counted the number of permutations with no matches; these are called derangements of \(D_n\). We will denote the number of permutations of \(D_n\) with exactly \(k\) matches by \(b_n(k) = \#\{N_n = k\}\) for \(k \in \{0, 1, \ldots, n\}\). In particular, \(b_n(0)\) is the number of derrangements of \(D_n\).
The number of derrangements is \[ b_n(0) = n! \sum_{j=0}^n \frac{(-1)^j}{j!} \]
By the complement rule for counting measure \(b_n(0) = n! - \#(\bigcup_{i=1}^n \{X_i = i\})\). From the inclusion-exclusion formula, \[ b_n(0) = n! - \sum_{j=1}^n (-1)^{j-1} \sum_{J \subseteq D_n, \; \#(J) = j} \#\{X_i = i \text{ for all } i \in J\} \] But if \(J \subseteq D_n\) with \(\#(J) = j\) then \(\#\{X_i = i \text{ for all } i \in J\} = (n - j)!\). Finally, the number of subsets \(J\) of \(D_n\) with \(\#(J) = j\) is \(\binom{n}{j}\). Substituting into the displayed equation and simplifying gives the result.
The number of permutations with exactly \(k\) matches is \[ b_n(k) = \frac{n!}{k!} \sum_{j=0}^{n-k} \frac{(-1)^j}{j!}, \quad k \in \{0, 1, \ldots, n\} \]
The following is two-step procedure that generates all permutations with exactly \(k\) matches: First select the \(k\) integers that will match. The number of ways of performing this step is \(\binom{n}{k}\). Second, select a permutation of the remaining \(n - k\) integers with no matches. The number of ways of performing this step is \(b_{n-k}(0)\). By the multiplication principle of combinatorics it follows that \(b_n(k) = \binom{n}{k} b_{n-k}(0)\). Using the result above for derrangements and simplifying gives the results.
The Probability Density Function
The probability density function of the number of matches is \[ \P(N_n = k) = \frac{1}{k!} \sum_{j=0}^{n-k} \frac{(-1)^j}{j!}, \quad k \in \{0, 1, \ldots, n\} \]
This follows directly from the result above on permutations with matches , since \(\P(N_n = k) = \#\{N_n = k\} \big/ n!\).
In the matching experiment, vary the parameter \(n\) and note the shape and location of the probability density function. For selected values of \(n\), run the simulation 1000 times and compare the empirical density function to the true probability density function.
\(\P(N_n = n - 1) = 0\).
A simple probabilistic proof is to note that the event is impossible—if there are \(n - 1\) matches, then there must be \(n\) matches. An algebraic proof can also be constructed from the probability density function of \( N_n \) above.
The distribution of the number of matches converges to the Poisson distribution with parameter 1 as \(n \to \infty\): \[ \P(N_n = k) \to \frac{e^{-1}}{k!} \text{ as } n \to \infty, \quad k \in \N \]
From the power series for the exponential function, \[ \sum_{j=0}^{n-k} \frac{(-1)^j}{j!} \to \sum_{j=0}^\infty \frac{(-1)^j}{j!} = e^{-1} \text{ as } n \to \infty \] So the result follows from the probability density function of \( N_n \) above.
The convergence is remarkably rapid.
In the matching experiment, increase \(n\) and note how the probability density function stabilizes rapidly. For selected values of \(n\), run the simulation 1000 times and compare the relative frequency function to the probability density function.
The mean and variance of the number of matches could be computed directly from the distribution. However, it is much better to use the representation in terms of indicator variables. The exchangeable property is an important tool in this section.
\(\E(I_j) = \frac{1}{n}\) for \(j \in \{1, 2, \ldots, n\}\).
\(X_j\) is uniformly distributed on \(D_n\) for each \(j\) so \(\P(I_j = 1) = \P(X_j = x) = \frac{1}{n}\).
\(\E(N_n) = 1\) for each \(n\)
This follows from the previous result and basic properties of expected value.
Thus, the expected number of matches is 1, regardless of \(n\), just as when the sampling is with replacement .
\(\var(I_j) = \frac{n-1}{n^2}\) for \(j \in \{1, 2, \ldots, n\}\).
This follows from \(\P(I_j = 1) = \frac{1}{n}\).
A match in one position would seem to make it more likely that there would be a match in another position. Thus, we might guess that the indicator variables are positively correlated.
For distinct \(j, \, k \in \{1, 2, \ldots, n\}\),
- \(\cov(I_j, I_k) = \frac{1}{n^2 (n - 1)}\)
- \(\cor(I_j, I_k) = \frac{1}{(n - 1)^2}\)
Note that \(I_j I_k\) is the indicator variable of the event of a match in position \(j\) and a match in position \(k\). Hence by the exchangeable property \(\P(I_j I_k = 1) = \P(I_j = 1) \P(I_k = 1 \mid I_j = 1) = \frac{1}{n} \frac{1}{n-1}\). As before, \(\P(I_j = 1) = \P(I_k = 1) = \frac{1}{n}\). The results now follow from standard computational formulas for covariance and correlation.
Note that when \(n = 2\), the event that there is a match in position 1 is perfectly correlated with the event that there is a match in position 2. This makes sense, since there will either be 0 matches or 2 matches.
\(\var(N_n) = 1\) for every \(n \in \{2, 3, \ldots\}\).
This follows from the previous two results on the variance and the covariance of the indicator variables, and basic properties of covariance. Recall that \(\var(N_n) = \sum_{j=1}^n \sum_{k=1}^n \cov(I_j, I_k)\).
In the matching experiment, vary the parameter \(n\) and note the shape and location of the mean \( \pm \) standard deviation bar. For selected values of the parameter, run the simulation 1000 times and compare the sample mean and standard deviation to the distribution mean and standard deviation.
For distinct \(j, \, k \in \{1, 2, \ldots, n\}\), \(\cov(I_j, I_k) \to 0\) as \(n \to \infty\).
Thus, the event that a match occurs in position \(j\) is nearly independent of the event that a match occurs in position \(k\) if \(n\) is large. For large \(n\), the indicator variables behave nearly like \(n\) Bernoulli trials with success probability \(\frac{1}{n}\), which of course, is what happens when the sampling is with replacement .
A Recursion Relation
In this subsection, we will give an alternate derivation of the distribution of the number of matches, in a sense by embedding the experiment with parameter \(n\) into the experiment with parameter \(n + 1\).
The probability density function of the number of matches satisfies the following recursion relation and initial condition:
- \(\P(N_n = k) = (k + 1) \P(N_{n+1} = k + 1)\) for \(k \in \{0, 1, \ldots, n\}\).
- \(\P(N_1 = 1) = 1\).
First, consider the random permutation \((X_1, X_2, \ldots, X_n, X_{n+1})\) of \(D_{n+1}\). Note that \((X_1, X_2, \ldots, X_n)\) is a random permutation of \(D_n\) if and only if \(X_{n+1} = n + 1\) if and only if \(I_{n+1} = 1\). It follows that \[ \P(N_n = k) = \P(N_{n+1} = k + 1 \mid I_{n+1} = 1), \quad k \in \{0, 1, \ldots, n\} \] From the defnition of conditional probability argument we have \[ \P(N_n = k) = \P(N_{n+1} = k + 1) \frac{\P(I_{n+1} = 1 \mid N_{n+1} = k + 1)}{\P(I_{n+1} = 1)}, \quad k \in \{0, 1, \ldots, n\} \] But \(\P(I_{n+1} = 1) = \frac{1}{n+1}\) and \(\P(I_{n+1} = 1 \mid N_{n+1} = k + 1) = \frac{k+1}{n+1}\). Substituting into the last displayed equation gives the recurrence relation. The initial condition is obvious, since if \(n = 1\) we must have one match.
This result can be used to obtain the probability density function of \(N_n\) recursively for any \(n\).
The Probability Generating Function
Next recall that the probability generating function of \(N_n\) is given by \[ G_n(t) = \E\left(t^{N_n}\right) = \sum_{j=0}^n \P(N_n = j) t^j, \quad t \in \R \]
The family of probability generating functions satisfies the following differential equations and ancillary conditions:
- \(G_{n+1}^\prime(t) = G_n(t)\) for \( t \in \R\) and \( n \in \N_+ \)
- \(G_n(1) = 1\) for \( n \in \N_+\)
Note also that \(G_1(t) = t\) for \(t \in \R\). Thus, the system of differential equations can be used to compute \(G_n\) for any \(n \in \N_+\).
In particular, for \(t \in \R\),
- \(G_2(t) = \frac{1}{2} + \frac{1}{2} t^2\)
- \(G_3(t) = \frac{1}{3} + \frac{1}{2} t + \frac{1}{6} t^3\)
- \(G_4(t) = \frac{3}{8} + \frac{1}{3} t + \frac{1}{4} t^2 + \frac{1}{24} t^4\)
For \(k, \; n \in \N_+\) with \(k \lt n\), \[ G_n^{(k)}(t) = G_{n-k}(t), \quad t \in \R \]
This follows from differential equation for the PGF given above.
For \(n \in \N_+\), \[ \P(N_n = k) = \frac{1}{k!} \P(N_{n-k} = 0), \quad k \in \{0, 1, \ldots, n - 1\} \]
This follows from the previous result and basic properties of generating functions.
Examples and Applications
A secretary randomly stuffs 5 letters into 5 envelopes. Find each of the following:
- The number of outcomes with exactly \(k\) matches, for each \(k \in \{0, 1, 2, 3, 4, 5\}\).
- The probability density function of the number of matches.
- The covariance and correlation of a match in one envelope and a match in another envelope.
- Covariance: \(\frac{1}{100}\), correlation \(\frac{1}{16}\)
Ten married couples are randomly paired for a dance. Find each of the following:
- The mean and variance of the number of matches.
- The probability of at least 3 matches.
- \(\E(N_{10}) = 1\), \(\var(N_{10}) = 1\)
- \(\P(N_{10} \ge 3) = \frac{145\,697}{1\,814\,400} \approx 0.08030037\)
In the matching experiment, set \(n = 10\). Run the experiment 1000 times and compare the following for the number of matches:
- The true probabilities
- The relative frequencies from the simulation
- The limiting Poisson probabilities
- See part (a) of the previous problem .

IMAGES
VIDEO
COMMENTS
Prevention of sharps injury. General rule for passing all instruments. Do not obstruct surgeon's view of operative site. Scalpel passed in neutral zone. Either surgeon or surgical technologist hand is in the area, not both; observe cautiously. Needle holder. Pass; needle tip up and same side as surgeon's thumb. Study with Quizlet and memorize ...
Chapter 12 Matching Exercise 12.03. Prevention of sharps injury. Click the card to flip 👆. Neutral zone. Click the card to flip 👆. 1 / 30.
Exercise 12.4.9. When \ (n = 100\) and \ (r = -0.89\), is there a significant correlation? Explain. This page titled 12.3E: The Regression Equation (Exercise) is shared under a CC BY 4.0 license and was authored, remixed, and/or curated by OpenStax via source content that was edited to the style and standards of the LibreTexts platform; a ...
Question: Assignment: Chapter 12 Assignment Assignment Score: 37.77% Save Submit Assignment for Grading nd Study Tools Questions Exercise 12.03 Algo (Goodness of Fit Test) Question 1 of 6 ffers Hint(s) Check My Work (3 remaining) 2. tions 3. access Tips During the first 13 weeks of the television season, the Saturday evening 8:00 P.M. to 9:00 P.M. audience proportions
Exercise 12.3.4. Does the scatter plot appear linear? Strong or weak? Positive or negative? Figure \(\PageIndex{6}\) Answer. The data appear to have no correlation. 12.3E: Scatter Plots (Exercises) is shared under a CC BY license and was authored, remixed, and/or curated by LibreTexts.
ignment: Chapter 12 Assignment Exercise 12.03 Algo (Goodness of Fit Test) 4 Question 4 of 10 Hint(s) Check My Work During the first 13 weeks of the television season, the Saturday evening 8:00 P.M. to 9:00 P.M. audience proportions were recorded as ABC 30%, CBS 27\%, NBC 23\%, and Independents 20\%.A sample of 300 homes two weeks after a Saturday night schedule revision yielded the following ...
This page titled 12.2: Exercises is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by Laird C. Sheldahl via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.
Ex 2 - Lab assignment; Physio Ex Exercise 5 Activity 7; Physio Ex Exercise 5 Activity 4; Physio Ex Exercise 6 Activity 3; Related Studylists API. Preview text. 191 Microscopic Anatomy and Organization of Skeletal Muscle E X E R C I S E 12 Skeletal Muscle Cells and Their Organization into Muscles 1. Use the items in the key to correctly identify ...
Matching Exercise 12.03. Term. 1 / 20. nims. Click the card to flip 👆. Definition. 1 / 20. provides the model for coordinating the emergency response plans and common standards. Develops the LEMA.
Matching Activity. Match the term with the correct definition. Push your learning experience beyond the classroom with the chapter 12 matching activity in the Teaching companion website.
Biology document from Arizona State University, 3 pages, Name: Date: School: Facilitator: 12.03 Male Reproductive System Male Reproductive System Using your lesson notes, complete the following: 1. Match the description to the reproductive structure.
45. a) 8 8 had seen exactly one. b) 6 6 had only seen SW. This page titled 12.3: Chapter 3 Exercise Solutions is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by Darlene Diaz ( ASCCC Open Educational Resources Initiative) via source content that was edited to the style and standards of the LibreTexts platform ...
drape is upside down-feet and arm-boards are exposed. ask for half-sheets to cover exposed areas. while anesthesia is taking drape, surgical technologists right glove is touched. remove contaminated outer right glove and reglove. small foreign body/hair noted on drape. remove with hemostat, pass hemostat to circulator, cover area.
Free PDF of NCERT Solutions for Class 12 Maths Chapter 3 Exercise 3.2 prepared by expert Mathematics teacher at Mathongo.com as per CBSE (NCERT) books guidelines. Download our Class 12 Maths Chapter 3 Matrices Ex 3.2 Questions with Solutions to help you to revise complete Syllabus and Score More marks in your exams. Download the FREE PDF.
Exercise 12.3E. 18 Determine if a Sequence is Arithmetic. In the following exercises, write the first five terms of each sequence with the given first term and common difference. a1 = 11 and d = 7. a1 = 18 and d = 9. a1 = −7 and d = 4. a1 = −8 and d = 5. a1 = 14 and d = −9. a1 = −3 and d = −3. Answer.
Question: Exercise 12.03 Algo (Goodness of Fit Test) Question 1 of 6 Hint (s) Check My Work (4 remaining) During the first 13 weeks of the television season, the Saturday evening 8:00 P.M. to 9:00 P.M. audience proportions were recorded as ABC 30%, CBS 28%, NBC 23%, and Independents 19%. A sample of 300 homes two weeks after a Saturday night ...
Question: Save Submit Assignment for Grading Exercise 12.03 Algo (Goodness of Fit Test) Question 1 of Check My Work (Sremainit) During the first 13 weeks of the television season, the Saturday evening 8:00 PM to 9:00 px audience proportions were recorded as AFC 29%, cos 26%, NBC 2396, and Independents 22%. A sample of 300 homes two weeks after ...
Study with Quizlet and memorize flashcards containing terms like supine, lateral, prone and more.
Click for sound. 5:23. Revisions are matched using the Sheet No. Click the Sheet No. drop down for each Plan and select the Sheet No. for which it is a revision/update. In our example, it is very clear that A03 and A07 are updates to existing Plans because they are named and numbered the same. Do this for both Plans and then click OK.
Figure 12.3E. 6 12.3 E. 6. Answer. The data appear to have no correlation. This page titled 12.3E: Scatter Plots (Exercises) is shared under a CC BY 4.0 license and was authored, remixed, and/or curated by OpenStax via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon ...
The probability density function of the number of matches is P(Nn = k) = 1 k!n − k ∑ j = 0( − 1)j j!, k ∈ {0, 1, …, n} Proof. In the matching experiment, vary the parameter n and note the shape and location of the probability density function. For selected values of n, run the simulation 1000 times and compare the empirical density ...
Display skeletal muscle activity. EMG. Useful to diagnose DVT (deep venous thrombosis) Phleborheography. View internal structures. Endoscopy. Study with Quizlet and memorize flashcards containing terms like Useful to detect diabetic vessel abnormalities, End-tidal levels of carbon dioxide, 24-hour monitoring of ECG and more.